Visualizing Complex Data
Sharks, the S&P 500, and lévy flights
Posted on 02/16/2011 by jacobalonso
2
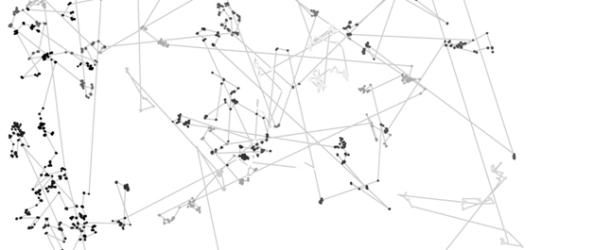
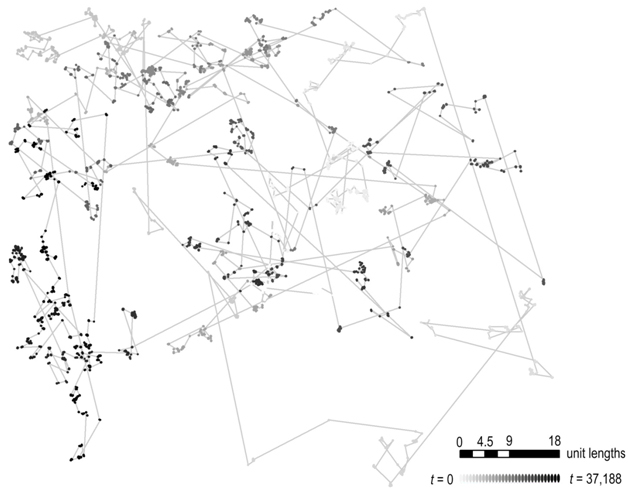
I have a particular fondness for mathematical concepts that describe large sets of natural phenomenon (like the oft-cited logarithmic spiral, which shows up in Nautilus shells, spiral galaxies, low pressure weather systems, and of course Romanesco broccoli). Something I came across while looking at the website of Paul Torrens for his work on empirical validation of agent-based behavioral models is this beautiful graphic:
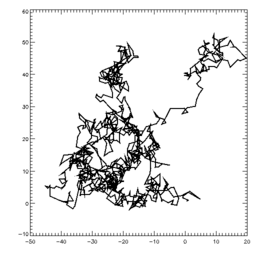
This is an illustration of a Lévy flight, essentially a mathematical description of a cluster of random short moves connected by infrequent longer ones. Lévy flights are described by a random walk, which is more or less any mathematical expression of a trajectory with successive random steps, distributed with a non-exponentially bounded probability distribution with theoretically infinite variance. So what does that mean? It is a description of motion. Take as a contrast Brownian motion, which is modeled after random movement of particles in a fluid.
A Lévy flight is instead a cluster of movements, some short and some long. It was identified by the late Benoit Mandelbrot, as an outgrowth of chaos theory, and has been applied to everything from foraging patters of animals to earthquakes (no really. See here).
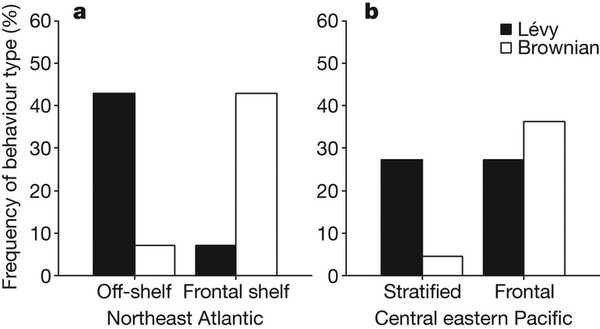
Take an animal foraging for food. A squirrely will stay within a small space in search of food, and then when it believes enough of the area has been searched, it will go in a random direction for a certain period, then begin foraging again. Similarly, sharks follow random Brownian motion when they are searching for food; however, if they cannot find any, they exhibit Lévy flight behavior, mixing short random movements with long trajectories. By the way, researchers discovered this by following a group of radio-tagged sharks for 5,700 days, collecting about 12 million movements (more here).
Previously, researchers had believed that albatross also followed Levy flights, but it now seems that they follow a much simpler random walk (explanation). Either way, it has been applied to pretty much anything you can image. Seth Godin even sees Lévy flights in social networks.
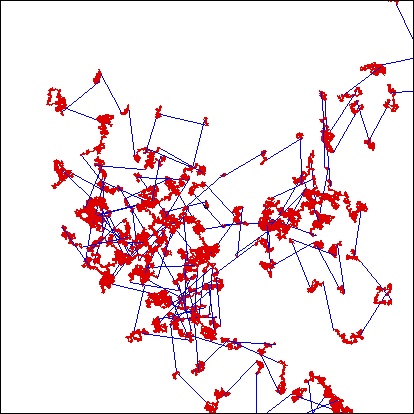
Michael Bigger explicates the idea, speculating that during the Vioxx scandal with Merck, a journalist finds the story and writes about it, which
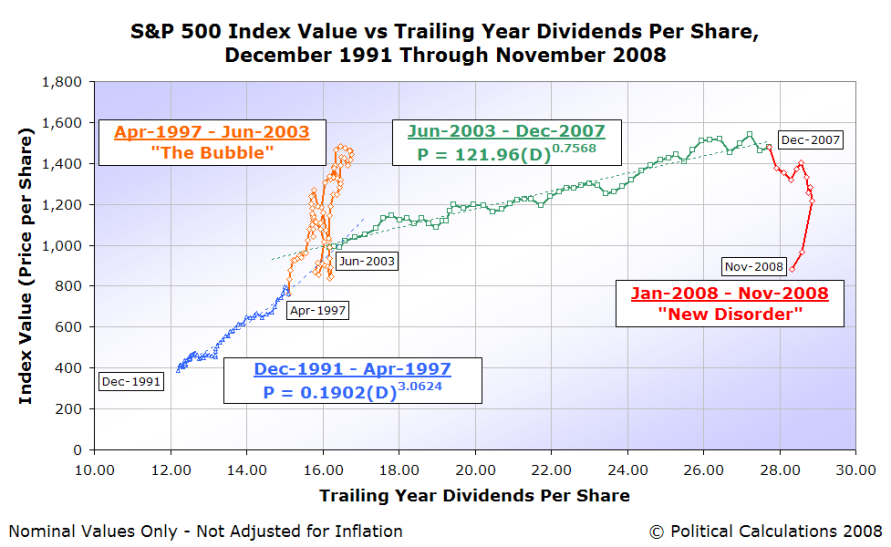
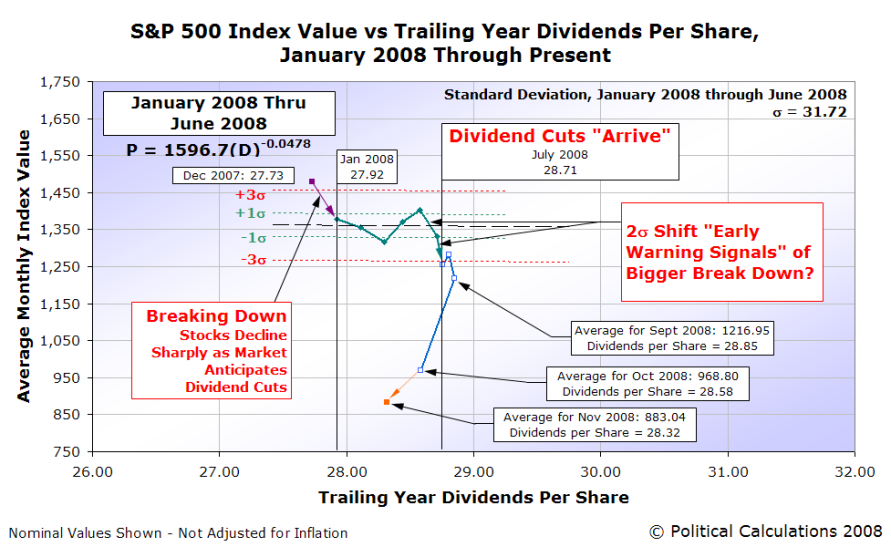
was big business news, and it stirred up emotions. Many people took a stance on both sides of the issues related to this event. Writing about Vioxx generated good readership and sold advertising. Eventually, readers got bored with the story and moved on. Our journalist had to find other news. The journalist’s path follows a Levy Flight as depicted in the image, from one random walk to a cluster, followed by the same process over and over again.On a similar note, the Political Calculations blog finds Lévy flights in financial markets (take a look here). Taking a look at long term S&P 500 dividends per share over a decade and a half, the authors argue that,
Since each data point represents approximately the same period of time, the number of trading days during a calendar month, we find that small movements predominate, which is consistent with the random walk behavior frequently observed in Brownian motion.Below is the long-term graph:
However, in the short-term since the financial crisis in 2008, there have been small, ‘normal’ random walks interspersed with large movements.
Comparing Brownian and Levy flight motion side-by-side as the authors do, you can get a better understanding of what they are talking about:

What I find so interesting about Lévy flights is their widespread application as a stochastic model in so many areas. I know that, at least for Brownian motion, the application is not necessarily meant to be exactly perfect, but rather, like a normal distribution, a general description of some behavior. I’m sure it can be applied in situations of micro-level crowd behavior, a la Paul Torrens. That would be something I would like to see—or has it been done already? Let me know.
Humphries, N., Queiroz, N., Dyer, J., Pade, N., Musyl, M., Schaefer, K., Fuller, D., Brunnschweiler, J., Doyle, T., Houghton, J., Hays, G., Jones, C., Noble, L., Wearmouth, V., Southall, E., & Sims, D. 2010. Environmental context explains Lévy and Brownian movement patterns of marine predators. Nature DOI:10.1038/nature09116
Thanks to:
https://seeingcomplexity.wordpress.com/2011/02/16/sharks-the-sp-500-and-levy-flights/